Page 17 - m4-unidad04

Página del Colegio de Matemáticas del Plantel 8 de la ENP-UNAM Sistemas de ecuaciones para modelar condiciones simultáneas Autor: Dr. José Manuel Becerra Espinosa

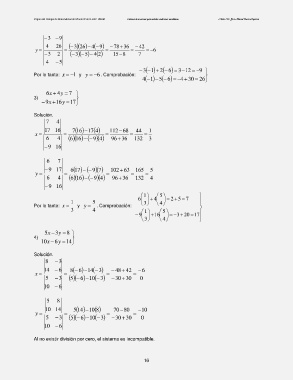
 3  9
4 26      94263    78 36  42
y       6
 3 2      2453   15 8 7
4  5

3    1 2 6 3 12  9 
Por lo tanto: x  1 y y   6 . Comprobación: 
4     1 5 6 4 30  26 

6x  4y  7 
3) 
9x 16y 17 

Solución.
7 4
17 16 7   1716    4 112 68 44 1
x     
6 4       49166   96 36 132 3
 9 16

6 7
 9 17 6      7917   102 63 165 5
y     
6 4       49166   96 36 132 4

 9 16
 1    5  
1 5  6  3   4  4   2  5  7 



Por lo tanto: x y y . Comprobación: 
3 4  1   5 
  9  16    3  20 17 
 3   4  


5x 3y  8 
4) 
10x 6y 14 

Solución.
8  3
14  6 8   146    3  48 42  6
x    
5  3    1065     3  30 30 0
10  6

5 8
10 14 5   1014    8 70  80  10
y    
5  3    1065     3  30  30 0
10  6

Al no existir división por cero, el sistema es incompatible.

16