Page 29 - m5-unidad02

Página del Colegio de Matemáticas del Plantel 8 de la ENP-UNAM Álgebra para analizar los objetos geométricos Autor: Dr. José Manuel Becerra Espinosa

2.4. ÁREA DE UN TRIÁNGULO

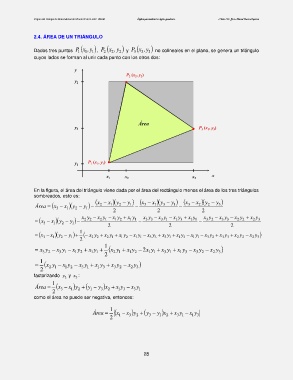
Dados tres puntos  ,xP 1 1 y 1  ,  ,xP 2 2 y 2  y  ,xP 3 3 y 3  no colineales en el plano, se genera un triángulo
cuyos lados se forman al unir cada punto con los otros dos:

En la figura, el área del triángulo viene dada por el área del rectángulo menos el área de los tres triángulos
sombreados, esto es:
x  x y  y  x  x y  y  x  x y  y 
Área  x  x y  y  2 1 2 1  3 1 3 1  3 2 2 3
1
3
2
1
2 2 2
x y  x y  x y  x y x y  x y  x y  x y x y  x y  x y  x y
 x  x y  y  2 2 2 1 1 2 1 1  3 3 3 1 1 3 1 1  3 2 3 3 2 2 2 3
3
2
1
1
2 2 2
1
 x  x y  y   x y  x y  x y  x y  x y  x y  x y  x y  x y  x y  x y  x y 
3
1
2
1
2 2 2 2 1 1 2 1 1 3 3 3 1 1 3 1 1 3 2 3 3 2 2 2 3
1

 x y  x y  x y  x y   yx  x y  2 yx  x y  x y  x y  x y 3
1
1
2
3
2
3
1
1
2 2 1 1 2 1 1 3 1 1 3 3 2 2
1
  yx 2 1  x 1 y  x 3 y  x 1 y  x 3 y  x 2 y 3 
2
2
3
1
2
factorizando y y x :
2
2
1
Área  x  x 1 y  y  y 3 x  x 1 y  x 3 y 1
3
2
1
2
3
2
como el área no puede ser negativa, entonces:

1
Área  x  x 3 y  y  y 1 x  x 3 y  x 1 y 3
2
3
2
1
1
2

28